
In these curved space geometries, shapes may be flat or curved within the space itself just as they could be in Euclidean geometry separate from the curvature of space itself. Opposed to the Euclidean geometries are the Non-Euclidean geometries (curved-space geometries) such as, for example, hyperbolic geometry and spherical geometry in which space curves to various degrees and in various ways. All of these multitude of geometries, in their utter complexities, are all extensions of Euclidean space geometry.

Though such higher geometries are impossible to visualize, mathematics can describe them. Euclidean space and its geometries can extend into three-dimensions (solid shapes such as polyhedrons), four-dimensions, and beyond with no limit (hyper-solids such as arbitrary polytopes). In the two-dimensional example of a piece of paper, the space itself is the piece of paper (not the shapes on them), and is Euclidean if on a flat desktop the space becomes non-Euclidean when the piece of paper is bent or curved or folded in on itself. But what distinguishes Euclidean space is the fact that the space itself does not curve. The shapes themselves may be curved, both in terms of its edges and its faces and such the shape itself may curve through space.
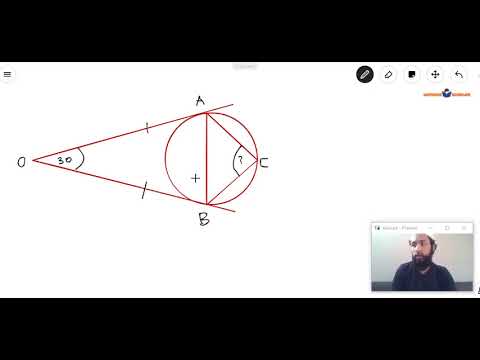
Shapes on a piece of paper, for example, such as in a high school geometry course, is and example of two-dimensional Euclidean geometry, or in other words geometry in two-dimensional Euclidean space. Euclidean space, and Euclidean geometry by extension, is assumed to be flat and non-curved. Euclidean geometry is, simply put, the geometry of Euclidean Space. It has its origins in ancient Greece, under the early geometer and mathematician Euclid.
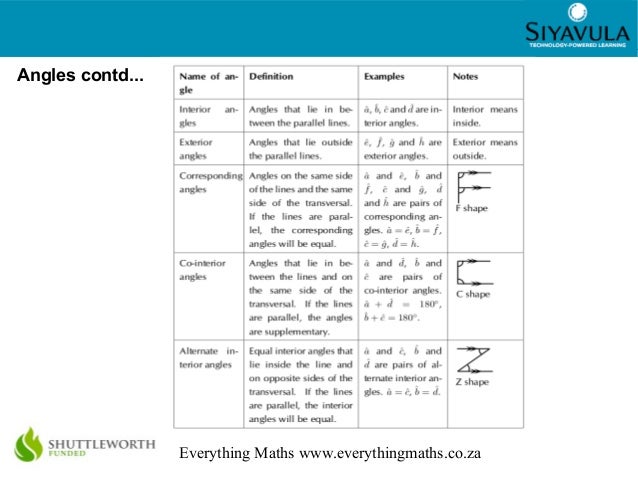
Euclidean geometry is a type of geometry that most people assume when they think of geometry.


 0 kommentar(er)
0 kommentar(er)
